A fairly standard CLV formula that you will find for measuring the lifetime value of existing customers is:
CLV = (m.r)/(1+d-r)
- Where m = customer margin (or profit contribution) per year
- Where d = discount rate
- And r = retention/loyalty rate per year
Important note: This formula is designed to measure the customer lifetime value of EXISTING customers only. You should also note that there is NO use of an acquisition cost, as existing customers have already been acquired and that expense is now historical (or a sunken cost).
How this CLV formula for existing customers works
To make sense of what this formula is doing, let’s first look at the bottom line of 1+d-r, but remove the discount rate (d) and we are now left with 1-r.
We should know that 1 minus the retention rate is churn (or customer loss) rate. When we use the churn rate at the bottom (denominator) of the fraction/equation, we are actually calculating the lifetime period of a customer in years.
- For example, a 60% loyalty rate = 40% churn/loss = 1/0.4 = 2.5 years, and
- An 80% loyalty rate = 20% churn/loss = 1/0.2 = 5 years
Now let’s look at the impact of the retention rate at the top of the equation. At the top of this CLV formula (numerator) there is m times r. This has the immediate impact of reducing the margin (profit) to the likely margin be be achieved in the FOLLOWING year.
This occurs because the formula is looking at an existing customer (already acquired) and estimating their FUTURE value – not their total value over the course of their customer relationship.
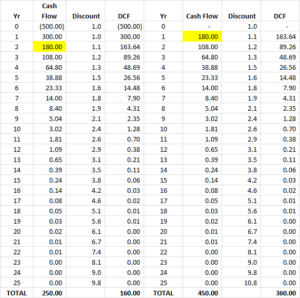
To make sense of this difference, let’s compare a new customer’s CLV to an existing customer.
The new customer is shown on the left hand side. As you can see, their acquisition cost was $500 in Year 0, their margin was $300 in Year 1, which then reduces by 60% each year in line with estimated retention rates.
After the 10% discount rate, their CLV (shown as DCF = discounted cash flow) is $160.
On the right hand side of the table, the CLV figures are shown for an existing customer using the above formula.
As you can see, there is NO acquisition cost (as they are already a customer), and we essentially start the customer in Year 2 with a annual margin of $180 (which is 60% of Year One’s $300 – note the two highlighted yellow cells).
In this case, the customer value (after discounting) is $360. This is also what the above formula tells us:
- CLV = (m.r)/(1+d-r) = (300 X 60%)/(1+.1-.6) = 180/0.5 = $360
Limitations of this CLV formula
The main concern with this approach to calculating customer lifetime value is its use of static values. Firstly, it assumes a stable margin (annual customer profit), which is generally unlikely (please see article on increasing customer revenues). And secondly, it also assumes a stable loyalty rate over time, which again is generally unlikely.
That’s why the free CLV Excel template available on this website allows for flexible revenues, costs and margins over time.
So why use this CLV formula?
CLV = (m.r)/(1+d-r) is appropriate as a simple estimation of future customer value. It can be easily applied to a customer database (say in a spreadsheet format) where the customer’s profit/margin for the year is listed, along with an estimated loyalty/retention rate.
This CLV value becomes a forward-looking metric that a marketer can use to determine the financial viability of various cross-selling and loyalty focused marketing tactics.
Related Topics
